コレクション graph of cylinder x^2 y^2=1 321014

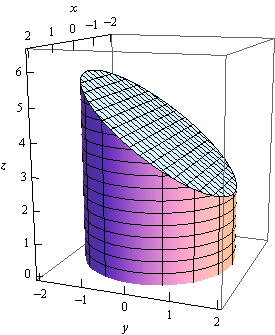
Let E be the region bounded below by the r θ r θplane, above by the sphere x 2 y 2 z 2 = 4, x 2 y 2 z 2 = 4, and on the sides by the cylinder x 2 y 2 = 1 x 2 y 2 = 1 (Figure 554) Set up a triple integral in cylindrical coordinates to find the volume of the region using the following orders of integration, and in each case findThe graph of a function f(x;y) = 8 x2 y) So, one surface we could use is the part of the surface z= 8 x 2 yinside the cylinder x2 y = 1 (right picture) 4 x y z x y z Let's call this surface Sand gure out how it should be oriented The original curve was parameterizedHow do you graph #x^2 y^2 = 1 #?

Question 5 Let The Surface S Be The Portion Of The Cylinder X2 Y2 4 Under Z 3 And Above The Xy Plane Write The Par Homeworklib

Graph of cylinder x^2 y^2=1

Graph of cylinder x^2 y^2=1-The steps to graph a circular cylinder with the z axis as the axis of symmetry, a radius of 2 units, a height of 3 units, and an origin of (x;y;z)=(0;0;0) are Step 1 Add a Graph application (page) to a document by selecting the Insert Graphs menu item Step 2 Change the graph from a 2D to a 3D graph by selecting the View 3D Graphing(e) Below is the graph of z = x2 y2 On the graph of the surface, sketch the traces that you found in parts (a) and (c) For problems 1213, nd an equation of the trace of the surface in the indicated plane Describe the graph of the trace 12 Surface 8x 2 y z2 = 9;

Find The Volume Of The Solid Bounced By The Planes Z 0 Z Y And X 2 Y 2 1 Mathematics Stack Exchange

It will be a sphere with radius = 1 unit As you know x^2y^2=1 is circle with radius = 1 Since here 3 coordinates x,y and Z are involved in the equation given by you, it will be 3d form of a circle which is obviously sphere you can understand it more clearly here Equation of a Sphere ExpiiSee the explanantion This is the equation of a circle with its centre at the origin Think of the axis as the sides of a triangle with the Hypotenuse being the line from the centre to the point on the circle By using Pythagoras you would end up with the equation given where the 4 is in fact r^2 To obtain the plot points manipulate the equation as below Given x^2y^2=r^2 >= 2t Find the equation of the plane through the point P and orthogonal to the line

Calculus questions and answers The graph of x2 2 = 1 is an ordinary right circular cylinder Indicate axis of the cylinder lies along and the radius of cylinder Use , between axis and radius Given the point P (4, 5, 1) and the line L x= 3 2t;Answer to 1 Find the point on the graph of y = x^{3} closest to the point (1, 0) Find the xvalue accurate to the nearest 01 2 A rightCylinder can be used as a geometric region and a graphics primitive Cylinder is equivalent to Cylinder { { 0, 0, 1 }, { 0, 0, 1 } } »

Now we draw the graph parametrically, as follows >The desired continuous graph will be created on the screen Here, continuous plot means that MATLAB marks each ordered pair (x i,y i), i=0,1,2,,n on the graph and then connects, by a straight line, the point (x 0,y 0) to (x 1,y 1), the point (x 1,y 1) to (x 2,y 2), and so on until the point (x n,y n) is reached This graph created with 11It is the equation of a circle Explanation Probably you can recognize it as the equation of a circle with radius #r=1#

Solved Try To Sketch By Hand The Curve Of Intersection Of The Parabolic Cylinder Y X 2 And The Top Half Of The Ellipsoid X 2 4y 2 4z 2 16 Then

Triple Integrals In Cylindrical And Spherical Coordinates

Plane z = 1 The trace in the z = 1 plane is the ellipse x2 y2 8 = 1EX 1 Sketch a graph of z = x2 y2 and x = y2 z2 5 A cylinder is the set of all points on lines parallel to l that intersect C where C is a plane curve and l is a line intersecting C, but not in the plane of C l 6 A Quadric Surface is a 3D surface whose equation is of the second degreeExtended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology &

Int Int B Int Dv Where B Is The Wedge Cut From The Cylinder X 2 Y 2 1 By The Planes Z 0 And Z Y Study Com

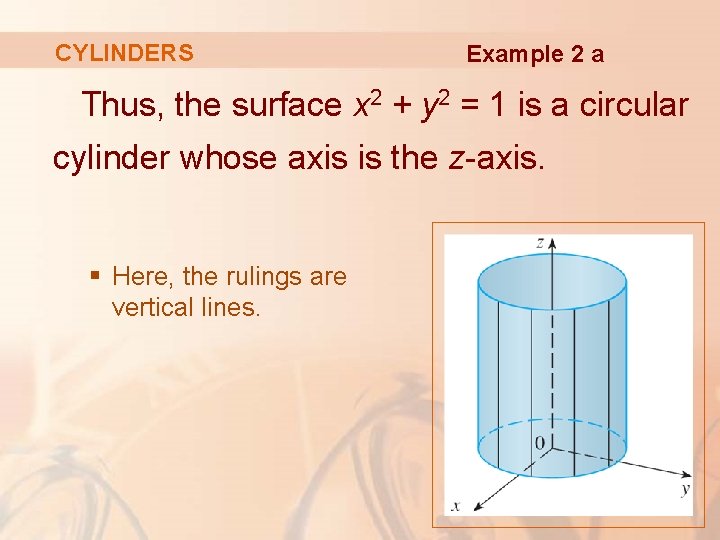
12 Vectors And The Geometry Of Space Vectors

Figure 8 Graph of the parabolic cylinder y = x2 in R3 Figure 9 Graph of the elliptic cylinder x2 y2 4 = 1 in R3 Example Describe the graph of x2 4 y2 16 = 1 Since z is a free variable, the trace in every horizontal plane z = k is a hyperbola Thus, the surface is a hyperbolic cylinder centered about the zaxisCylinderplot ( r,theta,sqrt (16r^2),r=04,theta=02*Pi);Cylinder represents a filled cylinder region where and the vectors are orthogonal with , and and Cylinder can be used in Graphics3D

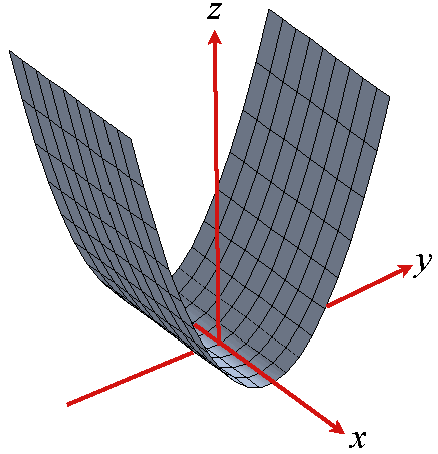
Level Surfaces

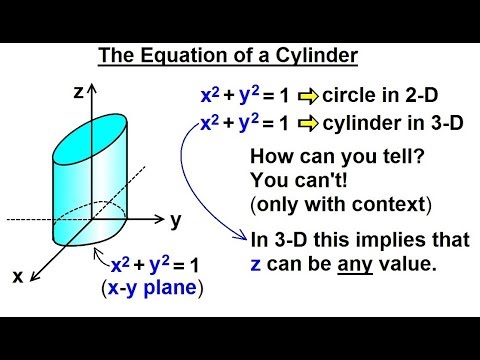
Calculus 3 Graphing In 3 D Basic Shapes 6 Of 9 The Equation Of A Cylinder Youtube

Sphere View interactive graph >Example 3611 Describe and sketch the surface x2 z2 =1 Whenever we are missing a variable in an equation, we know this will be a cylinder equation Let's first graph the equation if y =0 Remember that this is just the circle and not the interior Now, we consider if y could equal any value Then the picture changesThe cylinder ##y^2 z^2 = 1## has its central axis along the xaxis The bounding planes are x = 0 (the yz plane), z = 0 (the xy plane) and the plane y = x For this last plane draw the line y = x in the xy plane, and then extend the line up, keeping it parallel to the original line

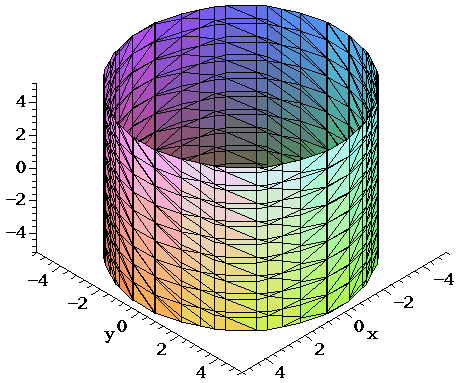
Surfaces Part 3

Triple Integrals In Cylindrical And Spherical Coordinates Calculus

Example 015 % The Viviani's Curve is the intersection of sphere x^2 y^2 z^2 = 4*a^2 %and cylinder (xa)^2 y^2 =a^2 %This script uses parametric equations for the Viviani's Curve,Z= k)x2 y2 k2 = 1 )x2 y2 = 1k2 The trace is a circle whose radius is p 1k2 Therefore the surface is a stack of circles, whose traces of other directions are hyperbola So it is a hyperboloid The intersection with the plane z= kis never empty This implies the hyperboloid is connected (b)If we change the equation in part (a) to x2 y2 z2 = 1, how is the graph168 Lagrange Multipliers Many applied max/min problems take the form of the last two examples we want to find an extreme value of a function, like V = x y z, subject to a constraint, like 1 = x 2 y 2 z 2 Often this can be done, as we have, by explicitly

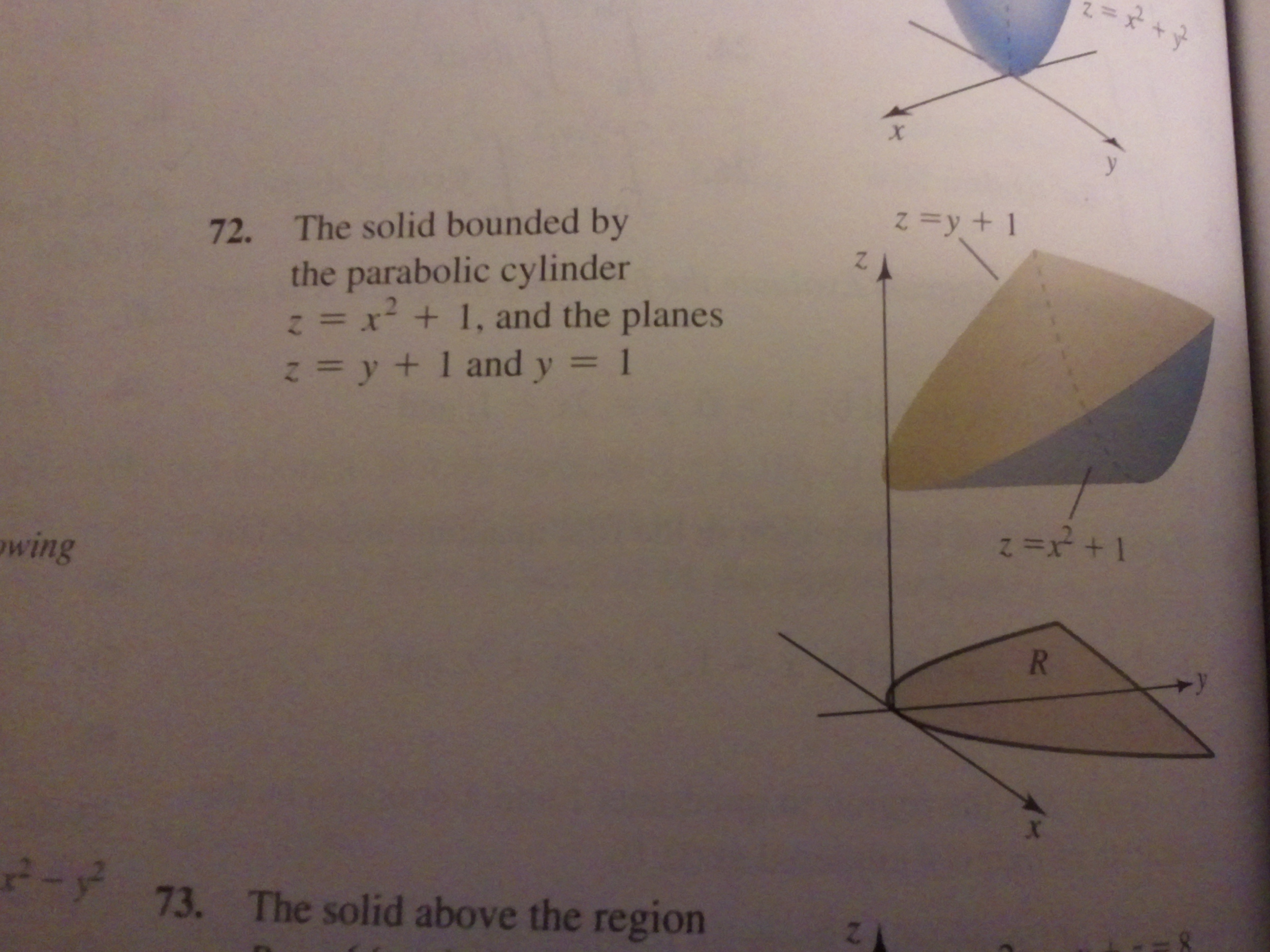
Solved What Is The Volume Of The Solid Bounded By The Chegg Com

Quadric Surfaces Calculus Volume 3

Math Input NEW Use textbook math notation to enter your math Try itLet W 1 be the solid halfcone bounded by z = p x 2 y 2, z = 1 and the yzplane with x ≥ 0, and let Let W 2 be the solid halfcone bounded by z = p x 2 y 2, z = 3 and the xzplane with y ≥ 0 For each of the following, decide (without calculating its value) whether the integral is positive, negative, or zero 2 ydV is ?/positiveCircleradiuscalculator radius x^2y^2=1 en Related Symbolab blog posts Practice, practice, practice Math can be an intimidating subject Each new topic we

Find A Vector Function That Represents The Curve Intersection Of The Two Surfaces The Cylinder X 2 Youtube

How To Calculate The Volume Of The First Octant Solid Bounded By The Cylinders X 2 Y 2 4 And X 2 Z 2 4 Quora

In this video explaining triple integration exampleFirst set the limits and after integrate This is very simple and good example#easymathseasytricks #defi46 CHAPTER 1 VECTORS AND THE GEOMETRY OF SPACE Figure 123 Graph of x2 4 y2 9 z2 16 = 1 Hyperboloid of one sheet The standard equation is x 2 a2 y b2 z c2 = 1 The minus sign could also be in front of the –rst or second fractionVolume of a cylinder?

Solved Example 4 Find The Volume Of The Solid That Lies Chegg Com

Graphing Elliptic Cylinders Mathematics Stack Exchange

We have to be careful not to draw interpret it as an equation in 2space even though there is no z that just means it does not depend upon z If we sketch this graph in the xyplane (so z = 0, we obtain the parabola y = x2 Since there is no zvalue in the equation, we shallLevel surfaces For a function $w=f(x,\,y,\,z) \, U \,\subseteq\, {\mathbb R}^3 \to {\mathbb R}$ the level surface of value $c$ is the surface $S$ in $U \subseteqPrecalculus Geometry of an Ellipse Graphing Ellipses 1 Answer Gió

The Wedge Cut From A Cylinder X 2 Y 2 1 By The Planes Z Y And Z 0 1 Sketch The Region Of Integration And Find The Bounds 2

15 8 Triple Integrals In Spherical Coordinates Mathematics Libretexts

Next, let us draw the cylinder x^2 y^2 = 2 In this cylinder, the radius r is always 2 We let theta vary from 0 to 2*Pi as usual, and let z range from 0 to 4 to match the the height of the sphere that we just drewParameterize the surface S, being the part of the paraboloid z = 10 x 2 y 2 lying inside the cylinder (x 1) 2 y 2 = 4 Viewing S as a graph, we first project onto the xyplane to obtain the domain of the function the disk D of radius 2 centered at (1,0) Now parameterize D using modified polar coordinates (x,y) = (1 ucosv,usinv)Show Solution Okay, since we are looking for the portion of the plane that lies in front of the y z y z plane we are going to need to write the equation of the surface in the form x = g ( y, z) x = g ( y, z) This is easy enough to do x = 1 − y − z x = 1 − y − z Next, we need to determine just what D D is

Spherical Coordinates In Matlab

2

In multivariable calculus, a cylinderis a surface in threespace where one variable is allowed to run free For example, consider the equation `z=x^2` We can look at the graph of this equation in two space;Okay, are copies of the same ellipse in the plane Z equals K So it follows that the surface for X squared plus y scrape was for is an elliptic cylinder which has rulings parallel to the Z axis With this description, I can catch the graph of this surface We have our X, y and Z axes, and first I'll draw the ellipse in the X Y planeSurfaces and Contour Plots Part 4 Graphs of Functions of Two Variables The graph of a function z = f(x,y) is also the graph of an equation in three variables and is therefore a surfaceSince each pair (x,y) in the domain determines a unique value of z, the graph of a function must satisfy the vertical line test already familiar from singlevariable calculus

Question 5 Let The Surface S Be The Portion Of The Cylinder X2 Y2 4 Under Z 3 And Above The Xy Plane Write The Par Homeworklib

Graph The Cylinder X 2 Y 2 16 And The Sphere X 2 Y 2 Z 2 49 Together Using Maple And Find The Volume Outside The Cylinder And Inside The Sphere Study Com

1) Observe the graph of the region, the wedges cut from a cylinder {eq}x^2 y^2 = 1 {/eq} by the planes {eq}z = y\ \text{and}\ z = 0 {/eq} See full answer below Become a member and unlock allThe solid enclosed by the cylinder y=x^2 and the planes z=0 and yz=1 Explanation According to Excercise 21 \\textbf{Excercise 21} Excercise 21, the solid is the region bounded by z = 0 z=0 z = 0, y = x 2 y=x^2 y = x 2 and y z = 1 yz = 1 y z = 1 Create a free account to see explanationsSteps to graph x^2 y^2 = 4

Find The Volume Bounded By The Parabolic X 2 Y 2 Az And The Cylinder X 2 Y 2 A 2

1

Professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicStack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeVideo Transcript we have given X equals two y squared minus one And now we have to make the graph And then we then, Lord, the question So for this this is the table which we have to make for little mining the value off X and y se x 00 Then why I will be here

15 8 Triple Integrals In Spherical Coordinates Mathematics Libretexts

Find The Area Of The Paraboloid Z 1 X 2 Y 2 That Lies In The First Octant Study Com

Along the yaxis A graph of the cylinder x2 z2 = 1 is above Example Let us examine the level surfaces for the function f(x;y;z) = x2 y2 z2 The set of points satisfying x 2y z2 = cfor c>0 forms a surface called a hyperboloid of one sheet, soGraph x^2y^2=1 x2 y2 = 1 x 2 y 2 = 1 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2 Match the values in this circle to those of the standard form The variable r r represents the radius of the circle, h h represents the xoffset from theKnowledgebase, relied on by millions of students &

Surfaces Part 3

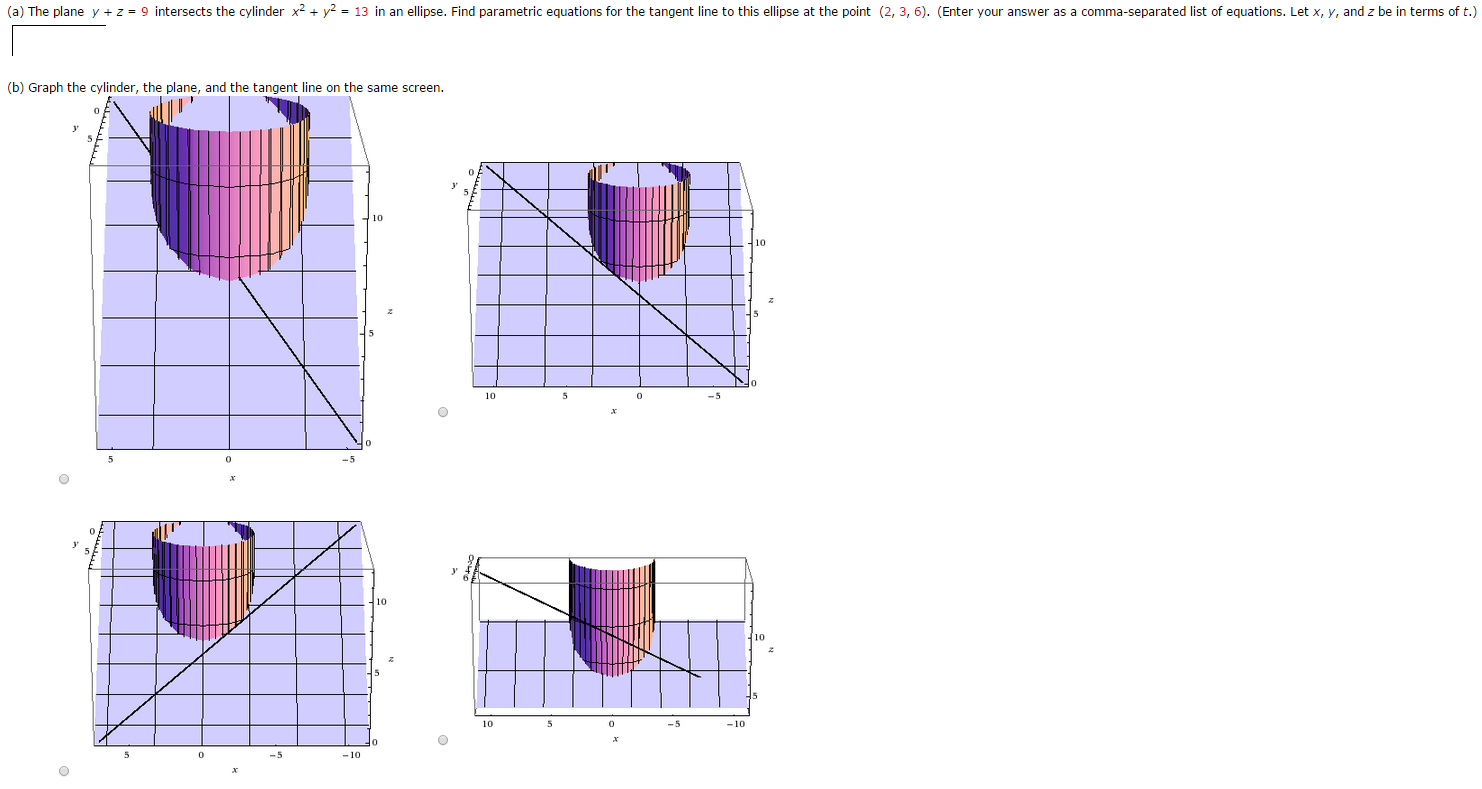
Solved A The Plane Y Z 9 Intersects The Cylinder X 2 Chegg Com

Traces are useful in sketching cylindrical surfaces For a cylinder in three dimensions, though, only one set of traces is useful Notice, in Figure 280, that the trace of the graph of z = sin x z = sin x in the xzplane is useful in constructing the graphThe trace in the xyplane, though, is just a series of parallel lines, and the trace in the yzplane is simply one lineFor example, if the equation is $(x1)^2 (y2)^2 = 4$, then one of the points on the cylinder is (1,0,0), but so is (1,0,1) and (1,0,1) and (1,0,5) and (1,0, 7) and so on This means that the cylinder goes on forever both up and downExample 12 Sketch the graph ofy = x2 (in 3space) Is it a cylinder?

Find The Volume Of The Solid Inside Both X 2 Y 2 Z 2 A 2 And X A 2 2 Y 2 A 2 2 Using A Triple Integral On Cylindrical Coordinates Mathematics Stack Exchange

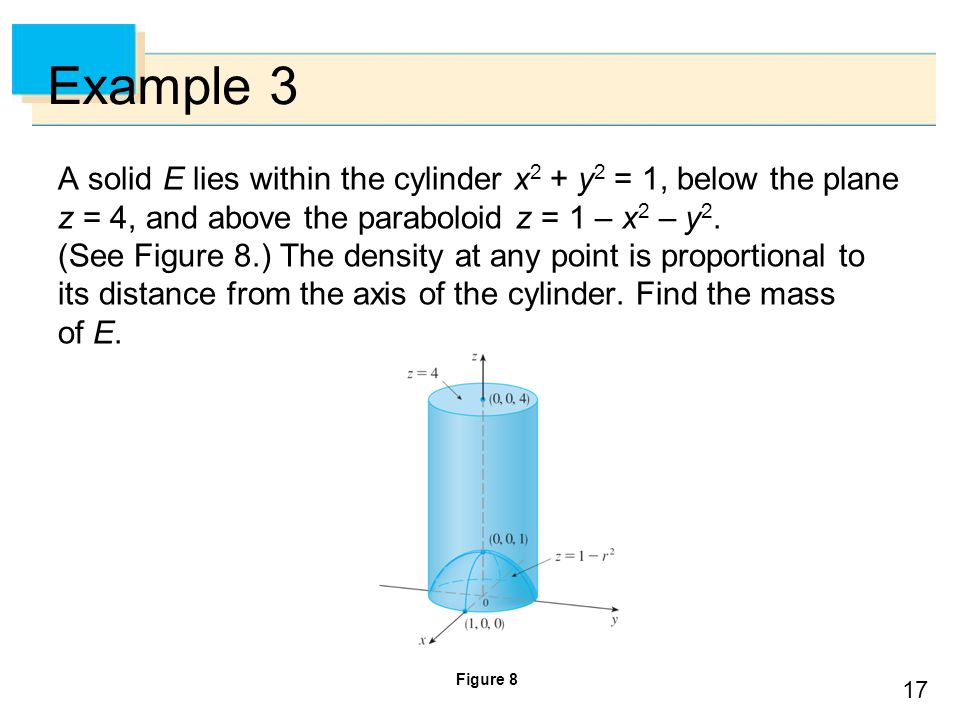
Copyright C Cengage Learning All Rights Reserved 15 Multiple Integrals Ppt Download

That is, we can graph `{(x,z)\ z=x^2}` The graph of this equation in the `xz`plane is showed in Figure 1Weekly Subscription $249 USD per week until cancelled Monthly Subscription $799 USD per month until cancelled Annual Subscription $3499 USD per year until cancelledWe want the equation describing the cylinder to be in its conventional form for simplicity We can subtract the 2 y from both sides and complete the square x 2 y 2 − 2 y = 0 We know that c = ( b 2) 2 so we can substitute that − 2 in the − 2 y term into the formula for b After substitution, we get that c = 1

How To Find The Volume Of The First Octant Section Cut From The Region Inside The Cylinder Math X 2 Z 2 1 Math By The Planes Math Y 0 Z 0 X Y Math Quora

23 Match The Equation X 2 Y 2 Z 2 1 With Its Graph Labeled I Viii Toughstem

MA 351 Fall 07 Exam #3 Review Solutions 4 6 Evaluate ZZ D x p y2 x2dA, D= f(x;y)j0 y 1;0 x yg ZZ D x p y2 x2dA= Z 1 0 Z y 0 x(y2 x2)12 dxdy (use usub on y2 x2) Z 1 0 1 3 (y2 x2)3=2 x=y x=0 dy= Z 1 0 1 3 (y2 y2)3=2 1 3 (y2)3=2 dy Z 1 0 1 12 y3 dy= 1 12 y4 1 0 = 1 12 7 Find the volume of the solid under the paraboloid z= x 2 y 4 and the planes x= 0, y= 0, z= 0 and x y= 1Algebra Graph x^2y^2=16 x2 y2 = 16 x 2 y 2 = 16 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2 Match the values in this circle to those of the standard form The variable r r represents the radius of the circle, h h represents the xoffset from the origin, and k k represents the yoffset fromIf one of the variables x, y or z is missing from the equation of a surface, then the surface is a cylinder Note When you are dealing with surfaces, it is important to recognize that an equation like x2 y2 = 1 represents a cylinder and not a circle The trace of the cylinder x 2 y = 1 in the xyplane is the circle with equations x2 y2

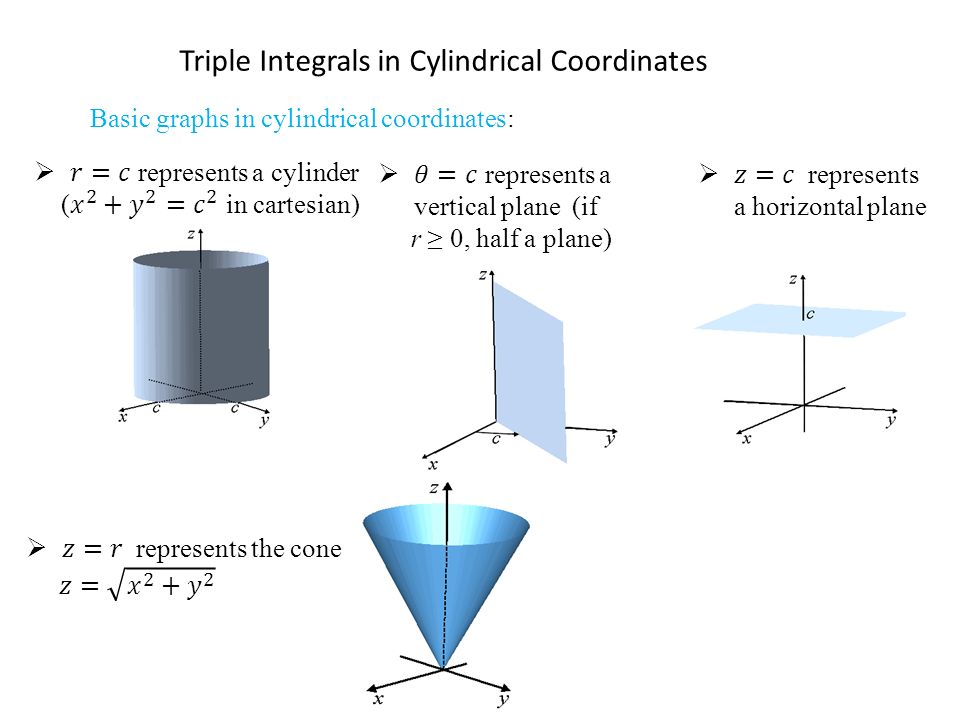
Triple Integral In Cylindrical Coordinates Ppt Video Online Download

What Is The Graph Of X 2 Y 2 Z 2 1 Quora

Piece of cake Unlock StepbyStep plot x^2y^2<1 and y>x Natural Language;

16 8 Lagrange Multipliers

Calculus Iii Surface Integrals

1

Calculus Part 2 Early Transcendentals 8th Edition 15 Pages 501 1000 Flip Ebook Pages 351 400 Anyflip Anyflip

Double Integrals In Polar Coordinates Calculus Volume 3

12 6 Quadric Surfaces Mathematics Libretexts

The Solid Enclosed By The Cylinder Y X 2 And The Planes Z 0 And Y Z 1 Youtube

14 6 Volume Between Surfaces And Triple Integration Chapter 14 Multiple Integration Part Calculus Iii

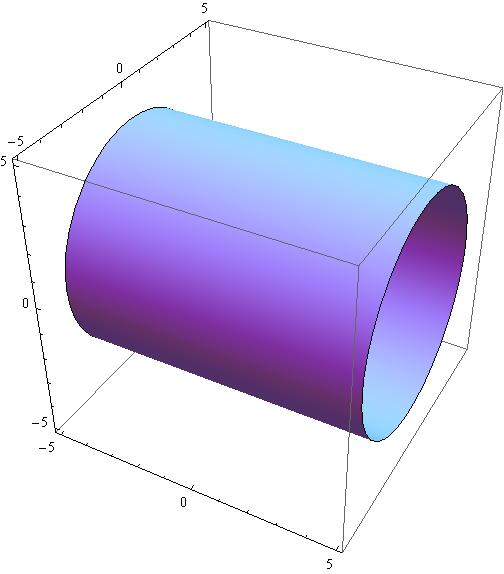
Y 2 Z 2 16 Is This Represents A Circle In 3 Dimensional Space Or 2 Dimensional Space Socratic

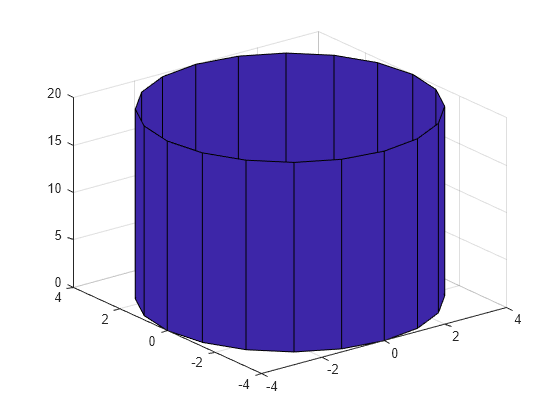
Create Cylinder Matlab Cylinder

Plotting In 3d

2

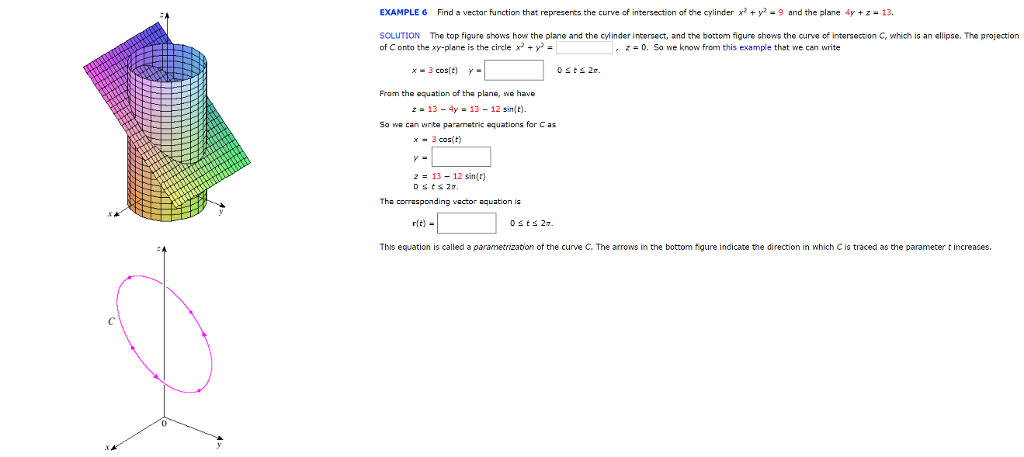
Solved Find A Vector Function That Represents The Curve Of Chegg Com

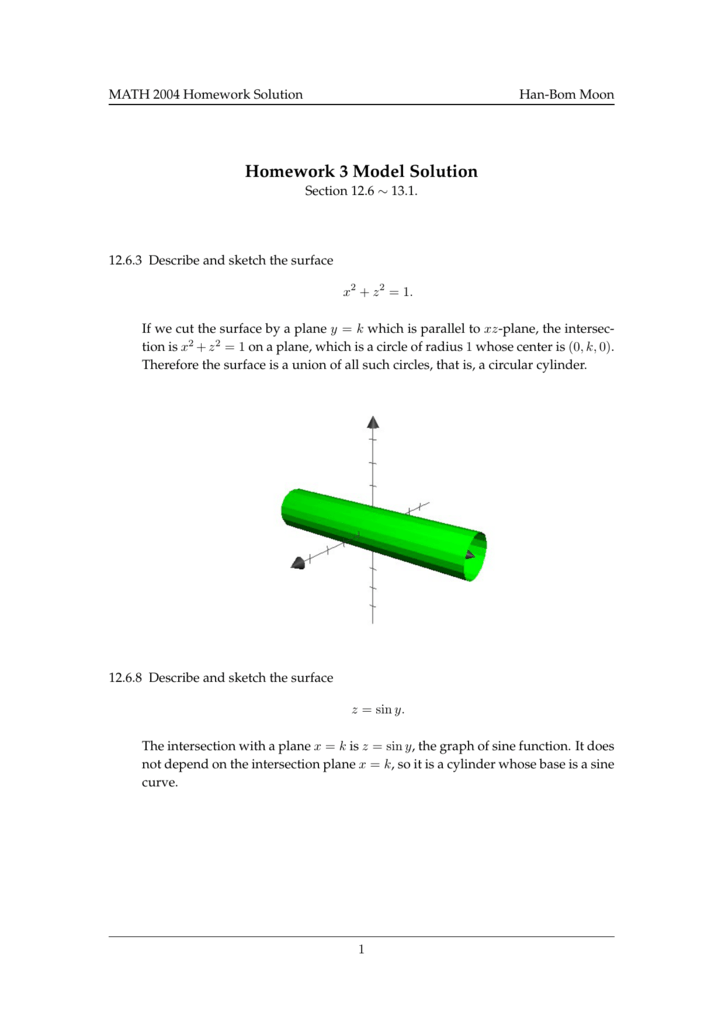
Homework 3 Model Solution Han

2

Find The Volume Of The Solid Bounced By The Planes Z 0 Z Y And X 2 Y 2 1 Mathematics Stack Exchange

Find The Volume Inside Both The Cylinder X 2 Y 2 1 And The Sphere X 2 Y 2 Z 2 4 Study Com

印刷可能 X2 Y2 Z21 Graph シモネタ

How Do I Plot X 2 Y 2 1 In 3d Mathematica Stack Exchange

15 8 Triple Integrals In Spherical Coordinates Mathematics Libretexts

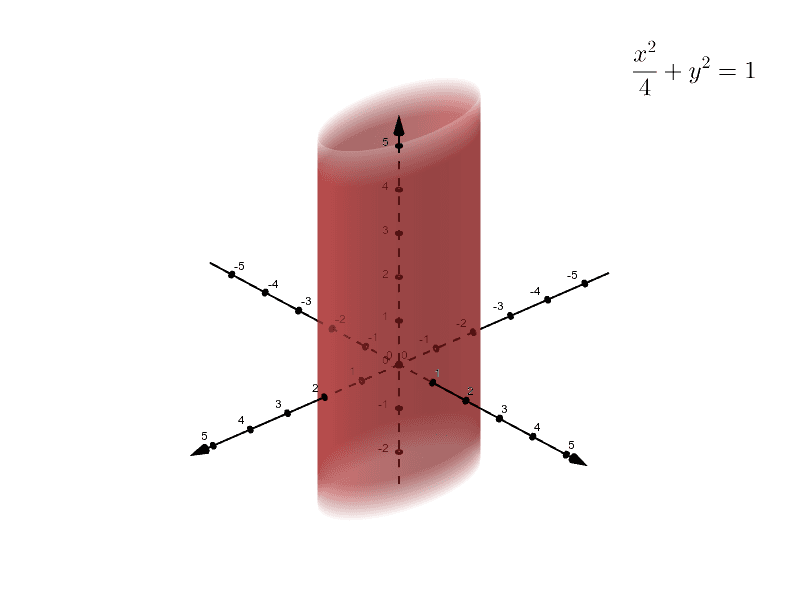
Cylinders And Quadric Surfaces Geogebra

Find The Area Of Part Of The Surface Z Xy That Lies Within The Cylinder X 2 Y 2 1 Study Com

How To Graph A Sphere With Cartesian Equations Mathematica Stack Exchange

12 6 Quadric Surfaces Mathematics Libretexts

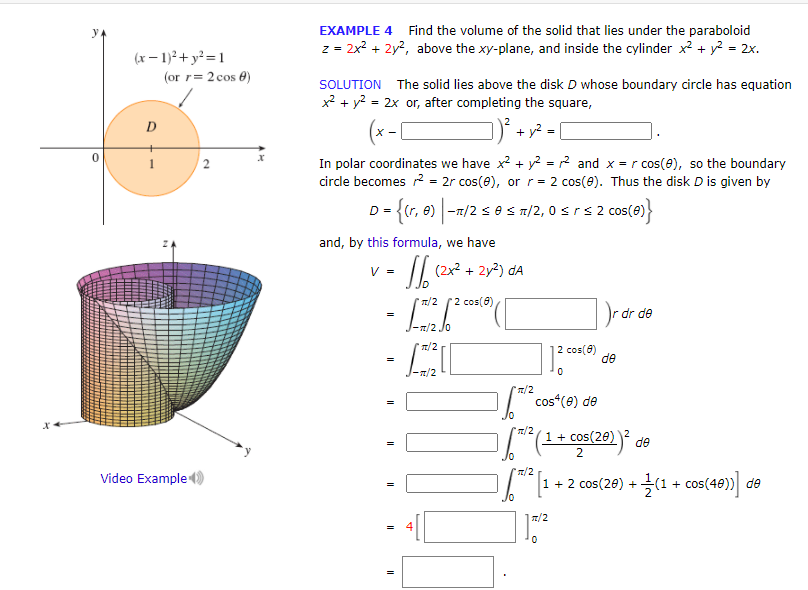
Solved Example 4 Find The Volume Of The Solid That Lies Chegg Com

2

How To Find The Volume Of The Solid Region That Is Bounded By The Cylinders X 2 Y 2 1 And X 2 Z 2 4 Quora

Thomas Calculus 11e 1263 1564 Pages 151 0 Flip Pdf Download Fliphtml5

Surface Area Of Z X 2 Y2 1 2 Youtube

Solved Find The Volume Of The Solid Bounded By The Graphs Of Chegg Com

Solved The Figure Shows The Surface Created When The Cylinder Y 2 Z 2 1 Intersects The Cylinder X 2 Z 2 1 Find The Area Of This Surface

3d Integration Cylindrical Coord

Find The Volume Inside Both The Cylinder X 2 Y 2 4 And The Ellipsoid 4x 2 4y 2 Z 2 Youtube

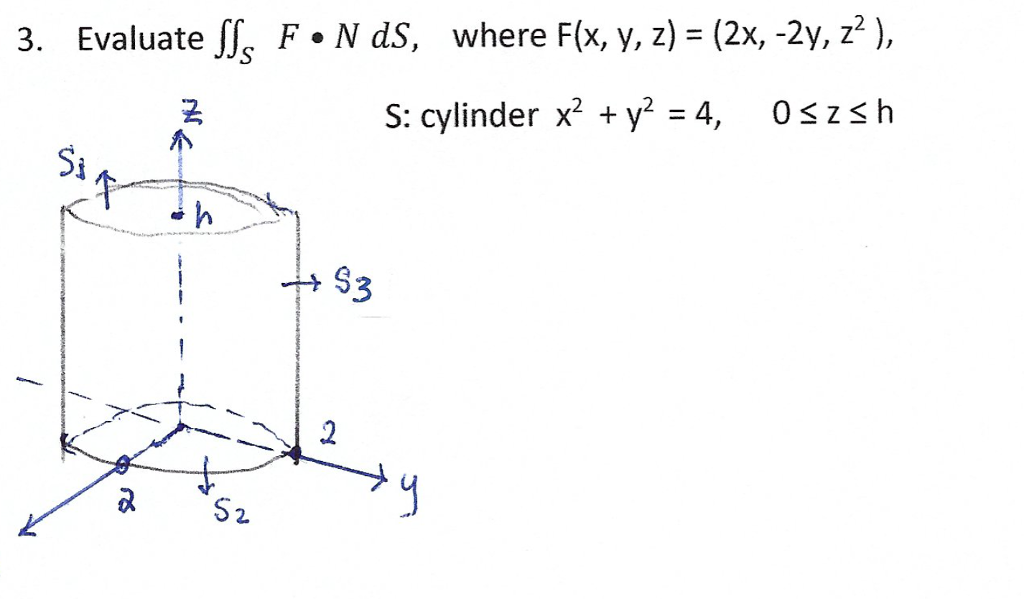
Solved Where F X Y Z 2x 2y S Cylinder X2 Y2 4 Chegg Com

1

Multiple Integrals H 2 Y Are Continuous Functions On C D And Let F X Y Be A Function Defined On R Then Pdf Free Download

1

Drawing Cylinders In Matlab

How Do I Plot X 2 Y 2 1 In 3d Mathematica Stack Exchange

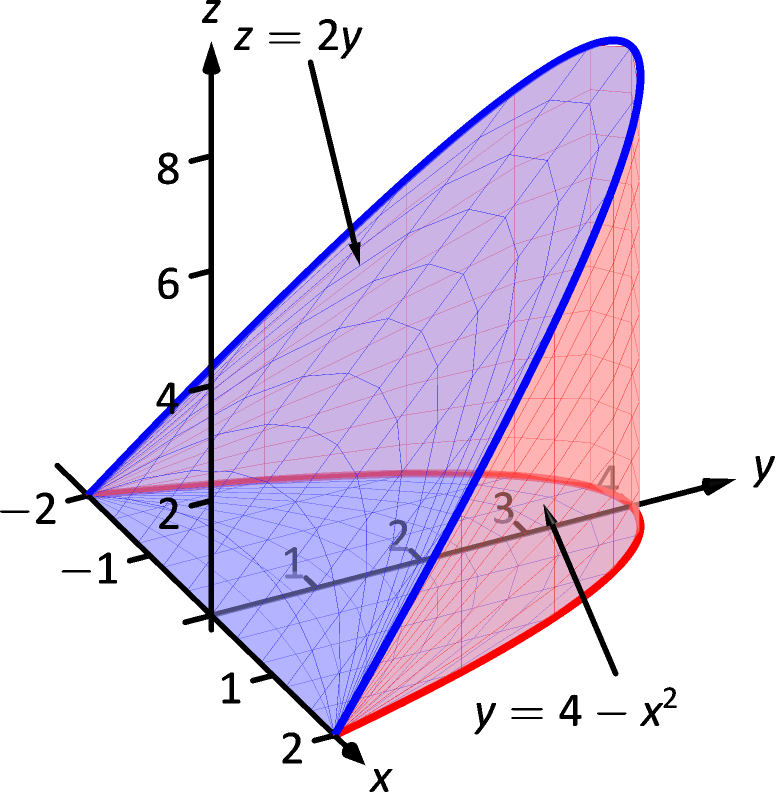
14 6 Volume Between Surfaces And Triple Integration Chapter 14 Multiple Integration Part Calculus Iii

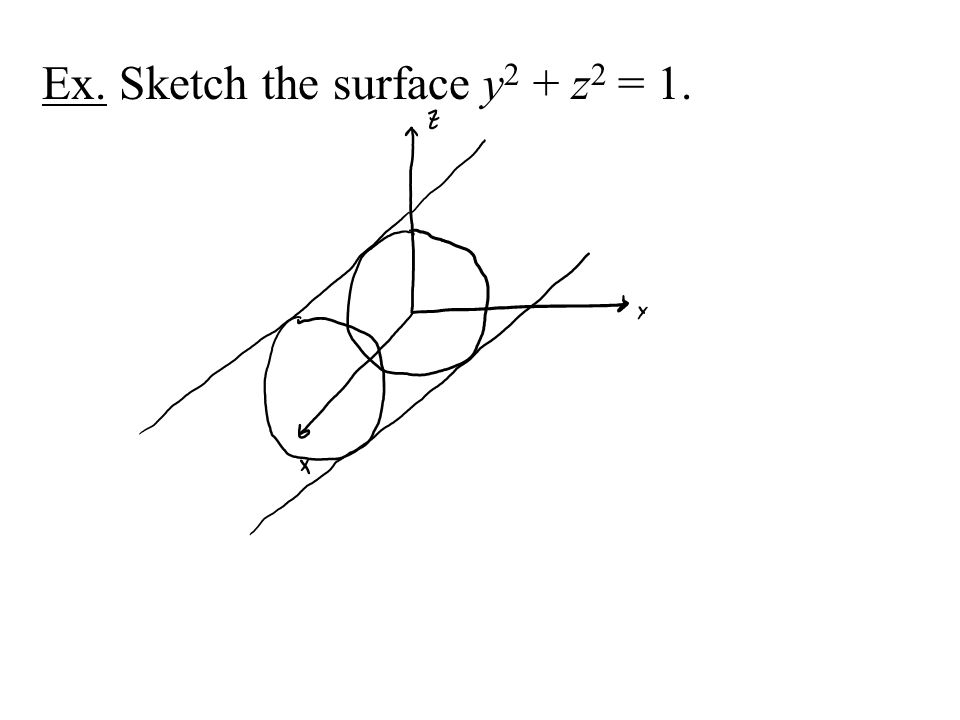
Cylinders And Quadratic Surfaces A Cylinder Is The Continuation Of A 2 D Curve Into 3 D No Longer Just A Soda Can Ex Sketch The Surface Z X Ppt Download

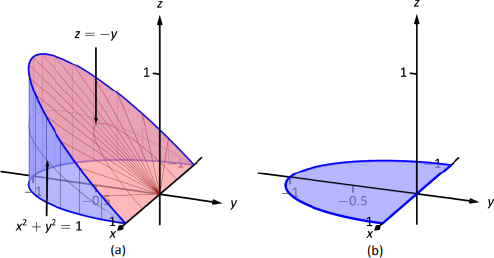
13 6 Volume Between Surfaces And Triple Integration Mathematics Libretexts

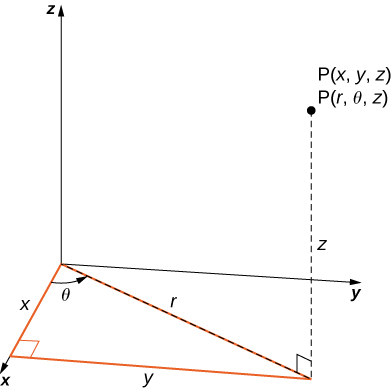
15 8 Triple Integrals In Spherical Coordinates Mathematics Libretexts

Level Surfaces

Find The Area Of The Surface The Part Of The Paraboloid Z X 2 Y 2 That Lies Inside The Cylinder X 2 Y 2 1 Study Com

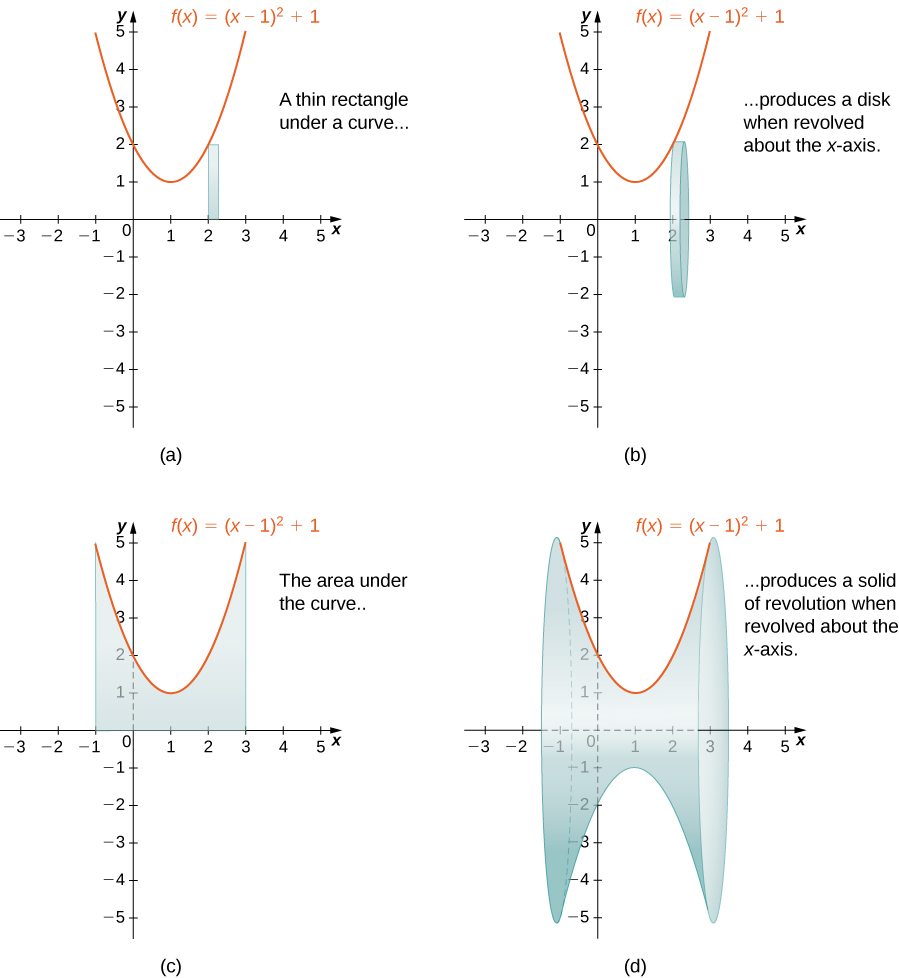
6 2 Determining Volumes By Slicing Calculus Volume 1

2

Surface Area

Plotting In 3d

What Is The Graph Of X 2 Y 3 X 2 2 1 Quora

Sec12 6 Html

Thomas Calculus 11e 1263 1564 Pages 151 0 Flip Pdf Download Fliphtml5

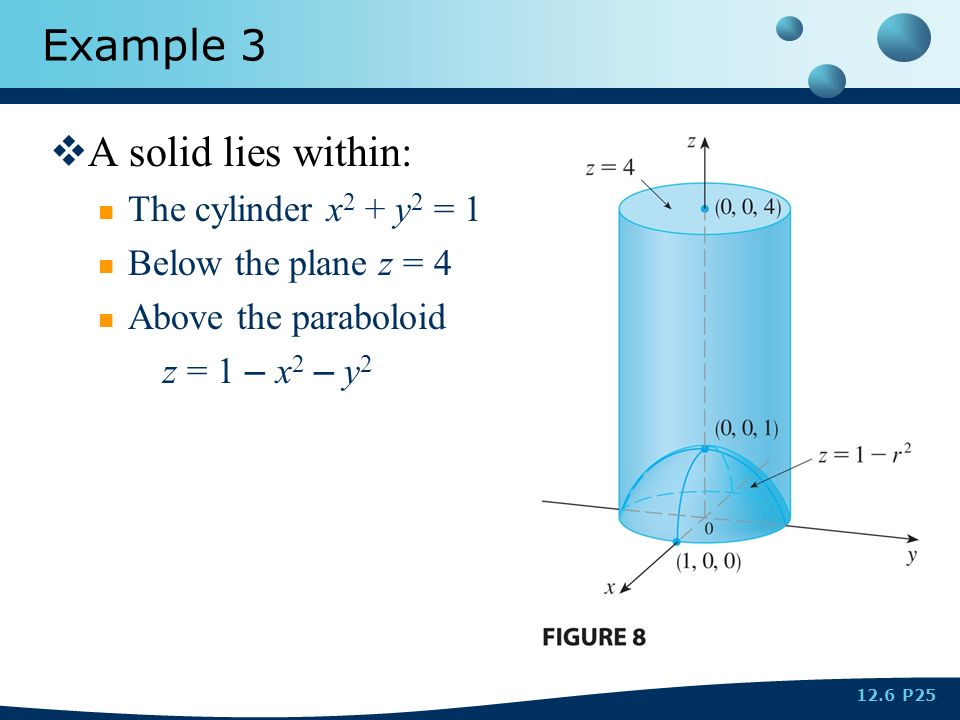
Section 12 6 Triple Integrals In Cylindrical Coordinates Ppt Download

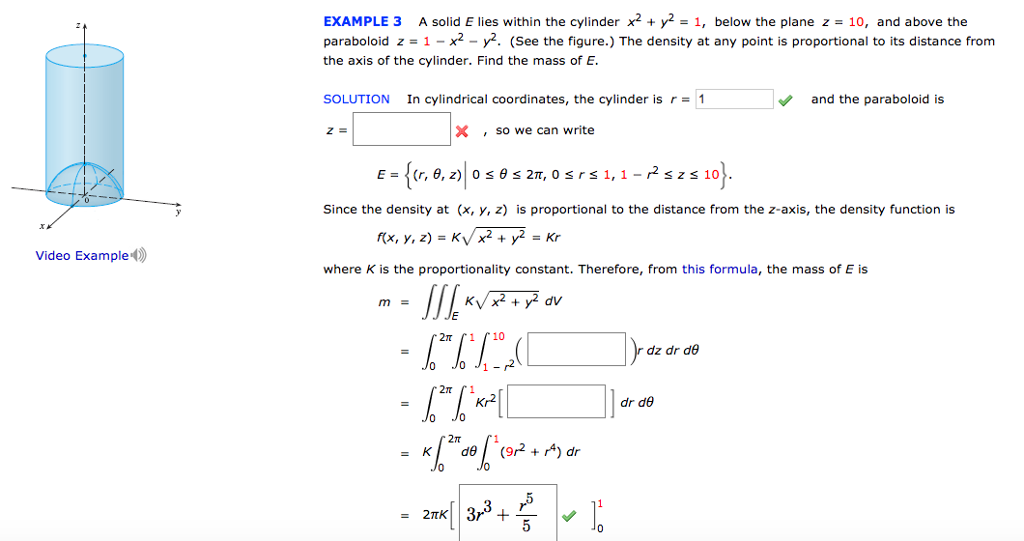
Solved A Solid E Lies Within The Cylinder X 2 Y 2 1 Chegg Com

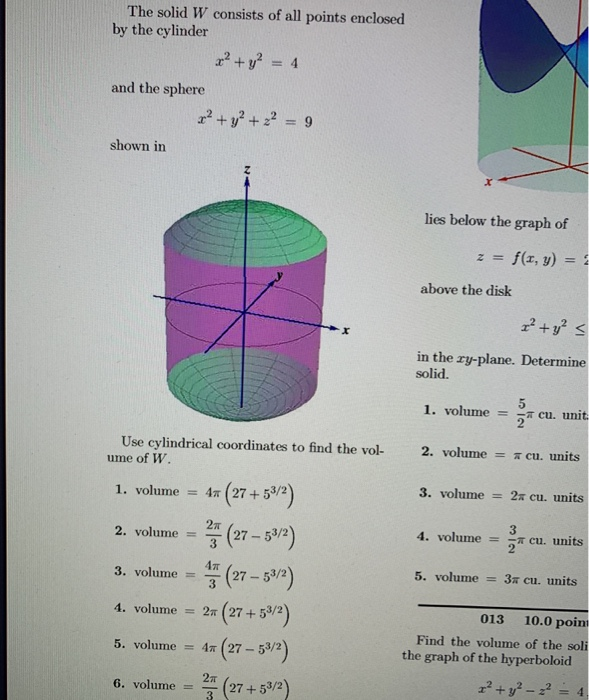
Solved The Solid W Consists Of All Points Enclosed By The Chegg Com

Find The Volume Of The Solid Inside The Cylinder X 2 Y 2 2ay And Bounded Above By The Sphere X 2 Y 2 Z 2 4a 2 And Below By The Xy Plane Study Com

A Paraboloid Described By Z X 2 Y 2 On The Xy Plane And Partly Inside The Cylinder X 2 Y 2 2y How

Triple Integral In Cylindrical Coordinates Ppt Video Online Download

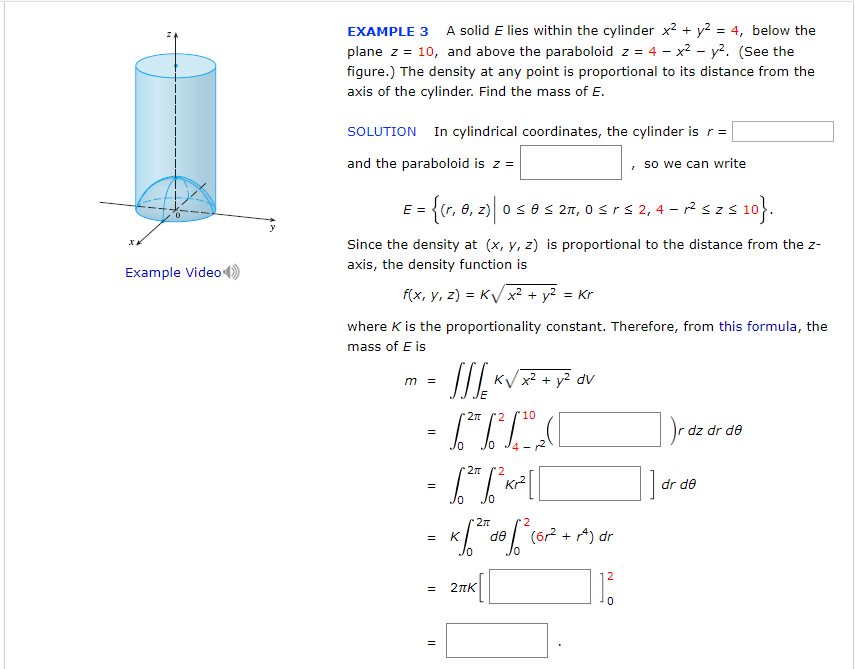
Solved Example 3 A Solid E Lies Within The Cylinder X2 Y2 Chegg Com

Simple Geometry Question Equation Of Cylinder Mathematics Stack Exchange

Solved If The First Cylinder Is X2 Y2 Where A Lt 1 Set Chegg Com

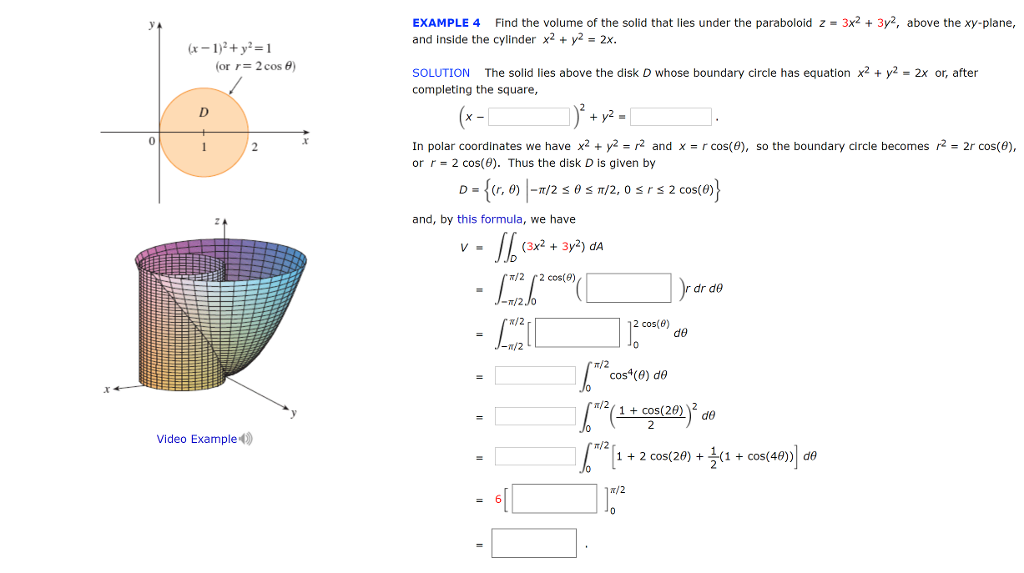
Solved Example 4 Find The Volume Of The Solid That Lies Chegg Com

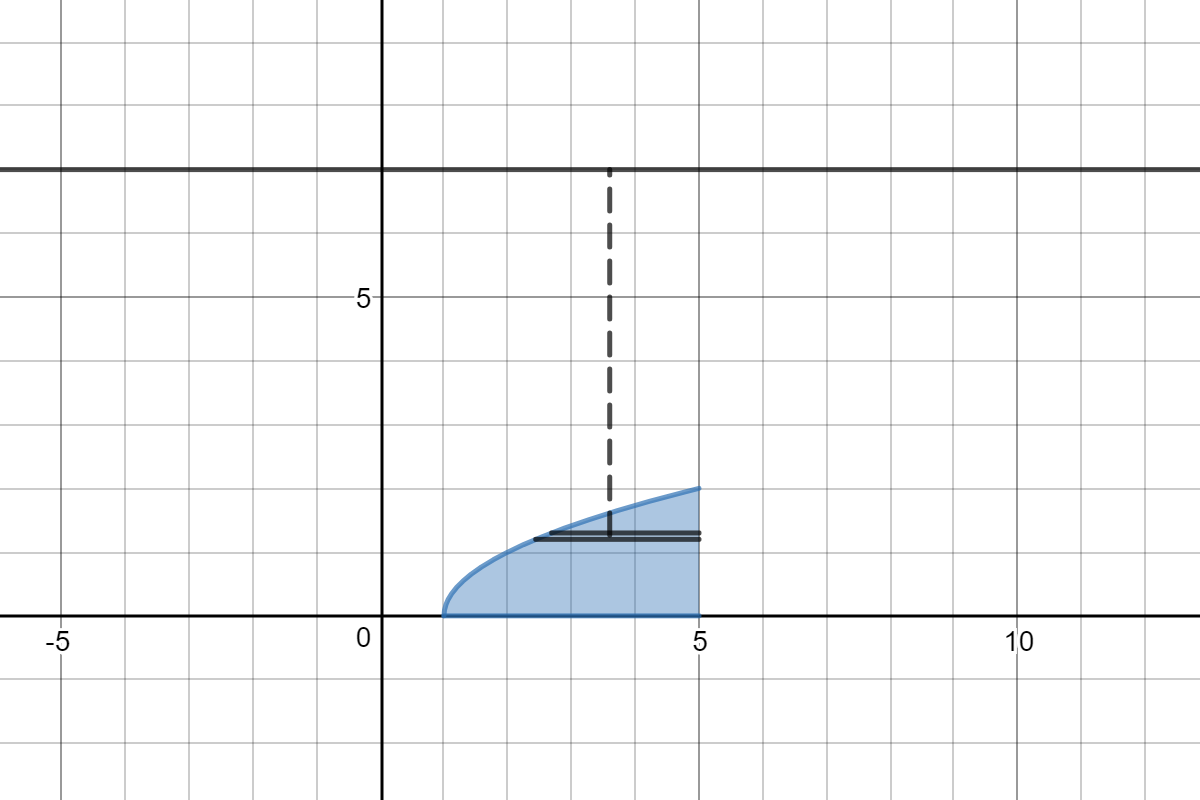
How Do You Find The Volume Of The Solid Obtained By Rotating The Region Bounded By Y Sqrt X 1 Y 0 X 5 Rotated About Y 7 Socratic

15 5 Parameterized Surfaces And Surface Area Chapter 15 Vector Analysis Part Calculus Iii

2

2

How Do You Graph X 2 Y 2 4 Socratic

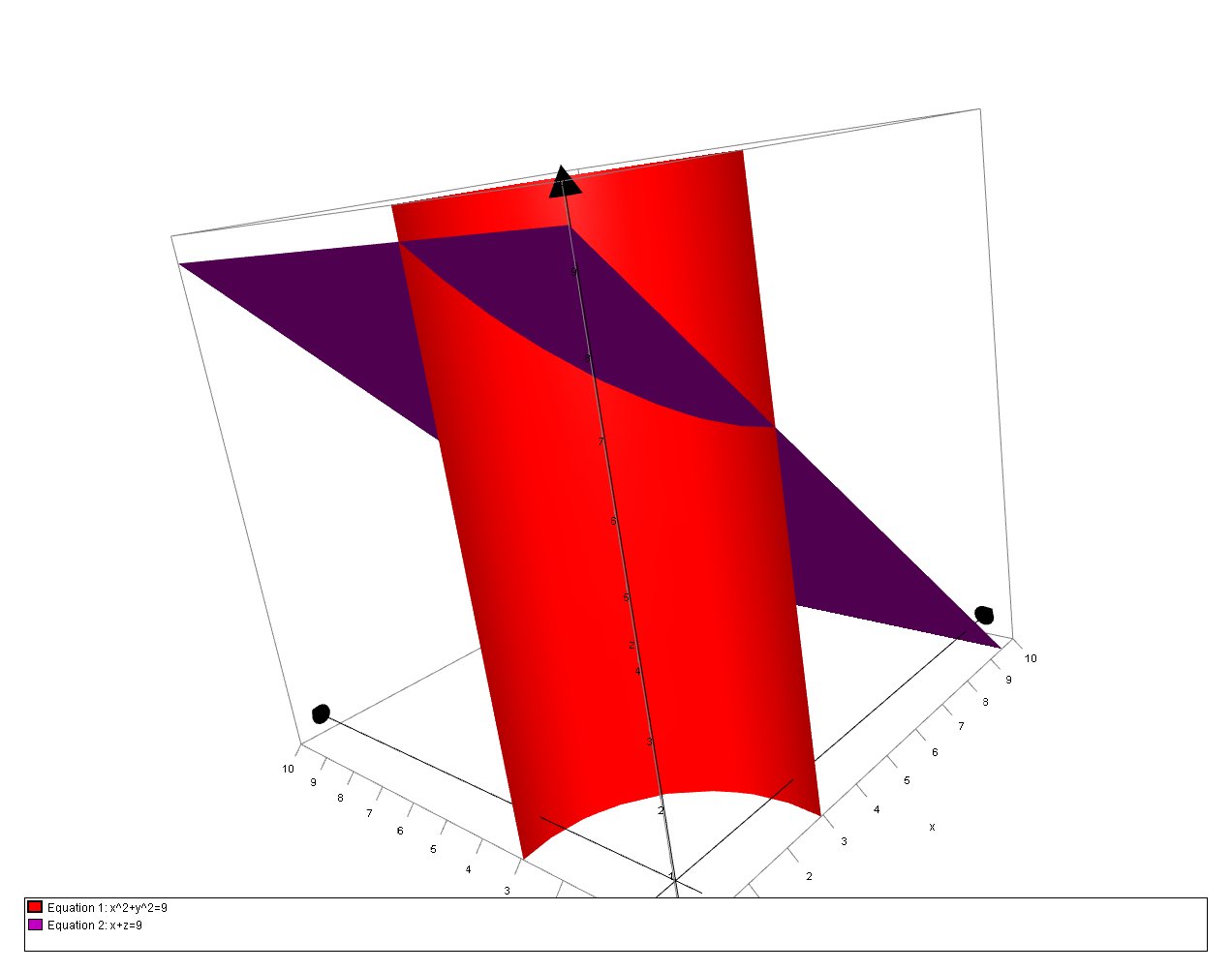
How Do You Find The Volume Of The Solid In The First Octant Which Is Bounded By The Coordinate Planes The Cylinder X 2 Y 2 9 And The Plane X Z 9 Socratic

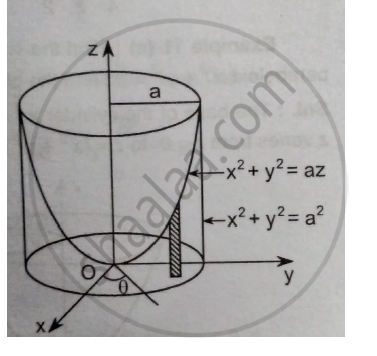
Find The Volume Bounded By The Paraboloid 𝒙𝟐 𝒚𝟐 𝒂𝒛 And The Cylinder 𝒙𝟐 𝒚𝟐 𝒂𝟐 Applied Mathematics 2 Shaalaa Com

009 Solid Geometry

Graph X 2 Y 2 4 Youtube

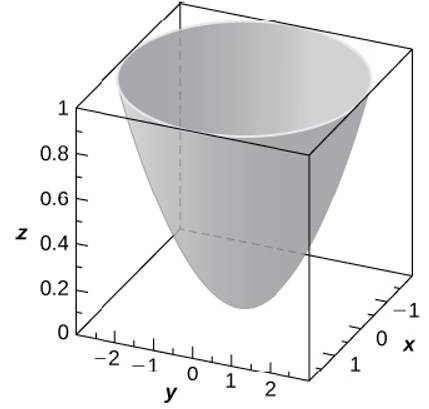
Use The Graph Of The Given Quadric Surface To Answer The Questions Specify The Name Of The Quadric Surface Which Of The Equations 36 Z 9 X 2 Y 2